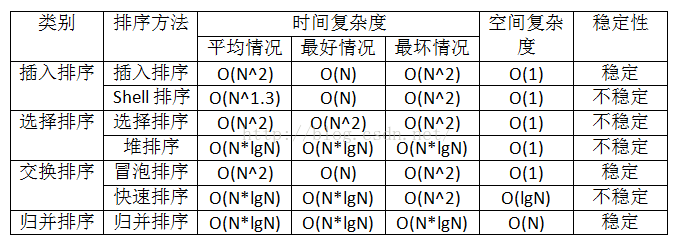
常用的排序算法
- 冒泡排序
/**
* 冒泡排序
* 时间复杂度:O(n*n)
* 空间复杂度:O(1)
* 稳定性:稳定
* 描述:通过与相邻的元素比较交换来把最小的元素交换到前面
*/
public class BubbleSort {
/**
* @param nums
*/
public static void bubbleSort(int[] nums){
// 最后一轮不用再比较
for (int i=0;i<nums.length-1;i++){
// 一轮过后最大的元素到了最后面
for (int j=0;j<nums.length-1-i;j++){
if (nums[j]>nums[j+1]){
int temp = nums[j];
nums[j] = nums[j+1];
nums[j+1] = temp;
}
}
}
}
/**
* 另一种写法
* 依次从数组中取一个数据,用这个数据和它之后的数据相比较
* @param nums
*/
public static void bubbleSort2(int[] nums){
for (int i=0;i<nums.length-1;i++){
// 一轮过后小的元素到最前面
for (int j=i+1;j<nums.length;j++){
if (nums[i]>nums[j]){
int temp = nums[j];
nums[j] = nums[i];
nums[i] = temp;
}
}
}
}
}
- 直接插入排序
/**
* 插入排序
* 时间复杂度:O(n*n)
* 空间复杂度:O(1)
* 稳定性:稳定
* 描述:对未排序数据,在已排序数据中从后向前扫描,找到相应位置并插入
*/
public class InsertionSort {
public static void insertionSort(int[] nums) {
int len = nums.length;
// 从第二个数开始
for (int i = 1; i < len; i++) {
// 选定一个元素
int key = nums[i];
// 扫描起始位置为已排序序列的末尾处
int j = i - 1;
// 扫描已排序序列,若元素比key大则将元素向后移
while (j >= 0 && nums[j] > key) {
nums[j + 1] = nums[j];
j--;
}
// 将选定的元素插入到查找到的位置
nums[j + 1] = key;
}
}
}
- 归并排序
/**
* 归并排序
* 时间复杂度:O(nlog2n)
* 空间复杂度:o(n)
* 稳定性:稳定
* 描述:分治法的思想,
* 先使每个子序列有序,再将已有序的子序列合并,
* 最后得到完全有序的序列;
*/
public class MergeSort {
public static void mergeSort(int[] nums){
sort(nums,0,nums.length-1);
}
// 分别对左右排序
public static void sort(int[] nums,int low,int high){
if (low==high){
return;
}
int mid = low + ( high - low ) / 2;
// 对左边排序
sort(nums,low,mid);
// 对右边排序
sort(nums,mid+1,high);
// 合并有序数组
merge(nums,low,mid,high);
}
// 合并
public static void merge(int[] nums,int low,int mid,int high){
// 暂时保存数组
int[] temp = new int[high-low+1];
int i = 0;
int l = low;
int m = mid+1;
// 从有序数组中挑选最小的放入temp中
while (l<=mid&&m<=high){
temp[i++] = nums[l]<nums[m]?nums[l++]:nums[m++];
}
// 处理未被放入的元素
while (l<=mid){
temp[i++] = nums[l++];
}
while (m<=high){
temp[i++] = nums[m++];
}
// 将排序后的序列放回原序列
System.arraycopy(temp,0,nums,low,temp.length);
}
}
- 选择排序
/**
* 选择排序
* 时间复杂度:O(n*n)
* 空间复杂度:O(1)
* 稳定性:不稳定
* 描述:在待排序序列找到一个最小的元素放到待排序序列的起始位置,再从剩余未排序序列重复相同操作
*/
public class SelectionSort {
public static void selectionSort(int[] nums){
for (int i=0;i<nums.length-1;i++){
int min = i;
// 找到最小值位置
for (int j=i+1;j<nums.length;j++){
if (nums[j]<nums[min]){
min = j;
}
}
// 将找到的最小值交换到待排序起始位置
int temp = nums[i];
nums[i] = nums[min];
nums[min] = temp;
}
}
}
- 希尔排序
/**
* 希尔排序
* 时间复杂度:O(n^3/2)
* 空间复杂度:O(1)
* 稳定性:不稳定
* 描述:插入排序的一种,又称缩小增量排序
* 把元素按下标的一定分量分组,对每组使用直接插入排序,
* 随增量的减少,每组包含的关键词越来越少,当增量减到1时,整个序列恰被分成一组,算法终止
*/
public class ShellSort {
public static void shellSort(int nums[]){
// 初始增量设为序列长
int gap = nums.length;
// 当增量为1时结束
while (gap!=1){
// 增量折半
gap/=2;
// 对分组做一次直接插入排序
for (int i=0;i<gap;i++){
for (int j=i+gap;j<nums.length;j+=gap){
int temp = nums[j];
int k = j-gap;
while (k>=0&&nums[k]>temp){
nums[k+gap] = nums[k];
k-=gap;
}
nums[k+gap] = temp;
}
}
}
}
}
- 快速排序
/**
* 快速排序
* 时间复杂度:O(nlog2n)
* 空间复杂度:O(nlog2n)
* 稳定性:不稳定
* 描述:一趟排序通过选取一个基准值,将要排序的数据分割成两部分,其中一部分所有元素都比另一部分小
* 再按此方法分别对两部分进行快速排序
*/
public class QuickSort {
public static void quickSort(int[] nums){
sort(nums,0,nums.length-1);
}
public static void sort(int[] nums,int low,int high){
int l = low;
int h = high;
// 选第一个元素为基准值,可以有多种取法,如果取值不好会导致时间复杂度变高
int key = nums[l];
// 一趟排序后已基准值为界分成两部分
while (l<h){
// 从前向后搜索,当大于key的值时停住
while (l<h&&nums[l]<key){
l++;
}
// 从后向前搜索,当小于key的值时停住
while (l<h&&nums[h]>key){
h--;
}
// 相等时不交换
if (nums[l]==nums[h]&&l<h){
l++;
}
else {
// 交换位置
swap(nums,l,h);
}
}
if (l-1>low){
// 对左边进行排序
sort(nums,low,l-1);
}
if (h+1<high){
// 对右边进行排序
sort(nums,l+1,high);
}
}
public static void swap(int[] nums,int i,int j){
int temp = nums[i];
nums[i] = nums[j];
nums[j] = temp;
}
}
7.堆排序
平常很少使用过堆排序,知道原理但是不会写。这次加了很多注释,记录一下。
/**
* 堆排序
* 时间复杂度:O(nlogn)
* 空间复杂度:O(1)
* 稳定性:不稳定
* 描述:堆是一个近似完全二叉树的结构,并同时满足堆积的性质:即子节点的键值或索引总是小于(或大于)它的父节点
* 堆排序的基本思想:将待排序的序列构造成一个大根堆,此时整个序列的最大值就是堆顶的根节点,
* 将其与末尾元素交换,此时末尾就为最大值.
* 然后将剩余n-1个元素重新构造成一个堆,再反复执行最终得到一个有序序列
*/
public class Heapsort {
public static void sort(int[] nums){
// 建堆
buildHeap(nums);
// 排序过程
for (int j=nums.length-1;j>0;j--){
// 将堆首交换至堆尾
swap(nums,0,j);
// 重新调整堆
adjustHeap(nums,0,j);
}
}
// 构建大根堆
static void buildHeap(int[] nums){
// 根据完全二叉树性质可推出,最后一个叶子节点为序列长度-1
// 从最后一个叶子节点开始,逐步向上调整堆
for (int i=nums.length/2-1;i>=0;i--){
adjustHeap(nums,i,nums.length);
}
}
// 调整大根堆
static void adjustHeap(int[] nums,int i,int length){
// 根节点
int temp = nums[i];
// i为根节点,则子节点为2*i+1和2*i+2
for (int k=2*i+1;k<length;k=2*k+1){
// 找左右子节点中较大的那一个,用于和父节点相比较
if (k+1<length&&nums[k]<nums[k+1]){
k++;
}
// 如果子节点大于根节点,则进行交换
if (nums[k]>temp){
swap(nums,i,k);
// 交换完成后,对以子节点为根的子树进行判断
i=k;
}
else {
// 不需要交换则这个子树是符合堆的性质的
break;
}
}
}
static void swap(int[] nums,int i,int j){
int temp = nums[i];
nums[i] = nums[j];
nums[j] = temp;
}
// 可以简易打印一棵较短的树
static void printTree(int[] nums){
int l = nums.length;
int n = 1;
int x = 2;
for (int num:nums){
for (int i = 0;i<l;i++){
System.out.print(" ");
}
System.out.print(num);
n++;
if (n>=x){
l = l/2;
x = x*2;
System.out.println();
}
}
System.out.println();
}
}